ERDC/CHL CETN-IV-27
September 2000
contours). The vector plot shows the direction of the increase or decrease. For example, in the
region of velocity magnitude increase just west of the spur, the vectors show the increase
directed at the point where the spur meets the east jetty. An increase implies that this alternative
may experience an increase in foundation scour in this area -- an unintended consequence of this
engineering alternative. Clearly, plots of this type can help identify consequences, both positive
and negative, caused by modifying existing configurations.
Sediment Transport
Often, hydrodynamic simulations are performed to gain insight into scour and deposition
patterns for a particular area. Rather than run a sediment transport model driven by the
hydrodynamic model output, innovative manipulation of hydrodynamic model output can
provide this insight without having to operate a sediment transport model. Primarily, shear stress
at the bed drives sediment transport. With velocity computed at peak ebb/flood as input, shear
stresses can be estimated in the following manner: assuming the Manning formula applies locally
(a reasonable assumption in working with the time scales of tidal flow which is quasi-steady
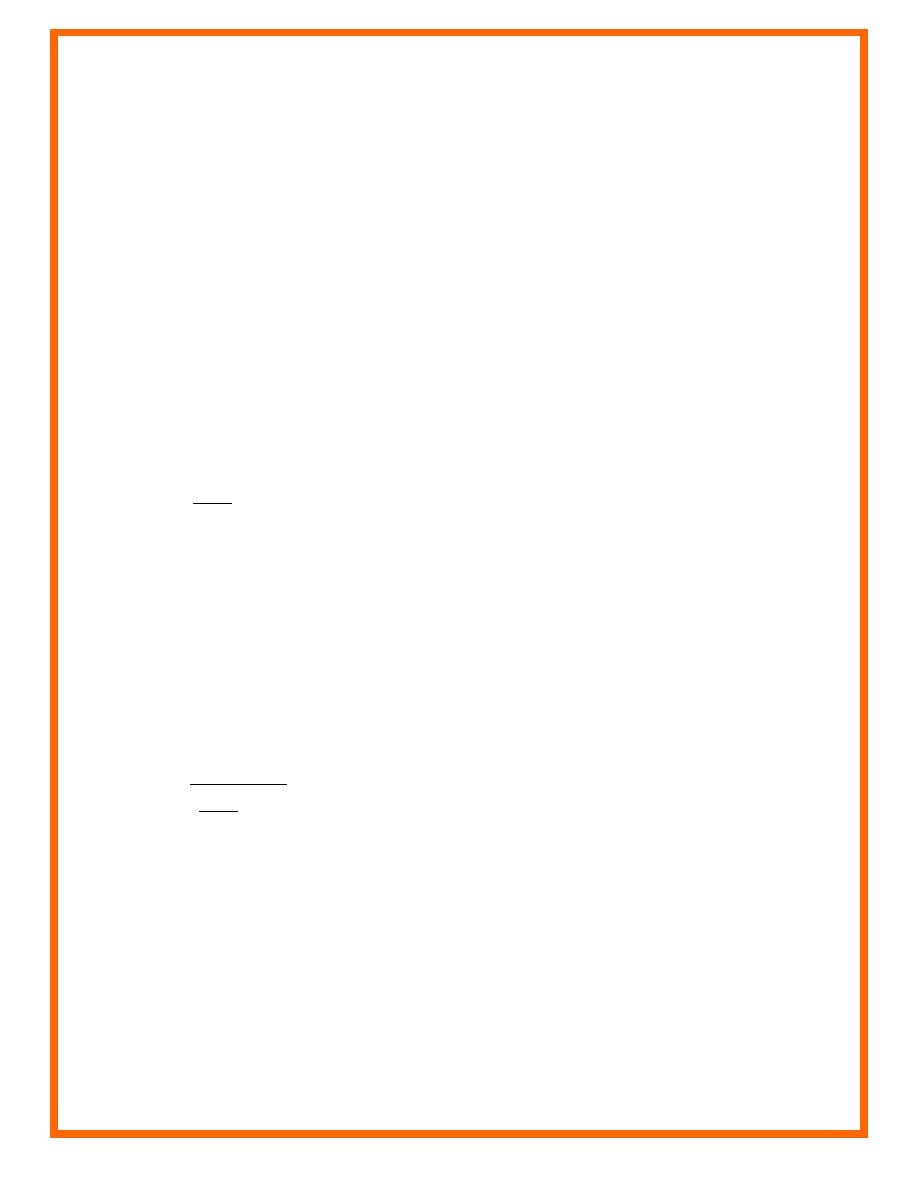
state), the Manning formula, in American customary units, is given by
1.486 2 / 3 1/ 2
V=
(1)
R S
n
where V is the depth-averaged velocity, n is Manning's n, R is the hydraulic radius (assumed to
be the local water depth), and S is the slope of the energy grade line. By momentum
conservation, for steady state flows, the following equation applies
τ = ρgRS
(2)
where τ is the shear stress at the bed, ρ is the mass density, g is gravity, R is the hydraulic radius,
and S is the slope of the energy grade line. Combining Equations 1 and 2 and eliminating the
slope of the energy grade line yields
ρgV 2
τ=
.
(3)
2
1.486 1/ 3
R
n
In regions of uniform Manning's n, creating plots of shear stress is possible within SMS.
Entering Equation 3 in the data calculator yields a scalar data set that SMS can plot (Figure 12).
To create vectors of shear stress, one must first assume that the shear stress acts in the direction
of the velocity. Next, the velocity vector data set is deconstructed into scalar data sets of velocity
direction and magnitude. Finally, the shear stress vector data set is constructed by combining the
shear stress magnitude scalar data set and the velocity direction scalar data set. The vectors in
Figure 12 are proportional to the shear stress magnitude.
11


 Previous Page
Previous Page
