ERDC/CHL CETN-IV-25
June 2000
energy cannot be transported against the current, and the waves are "stopped" (in the real case
this often implies confused wave conditions that could cause navigational hazards). The
criterion for determining the limit for wave blocking is given by (Jonsson, Skougaard, and Wang
1970):
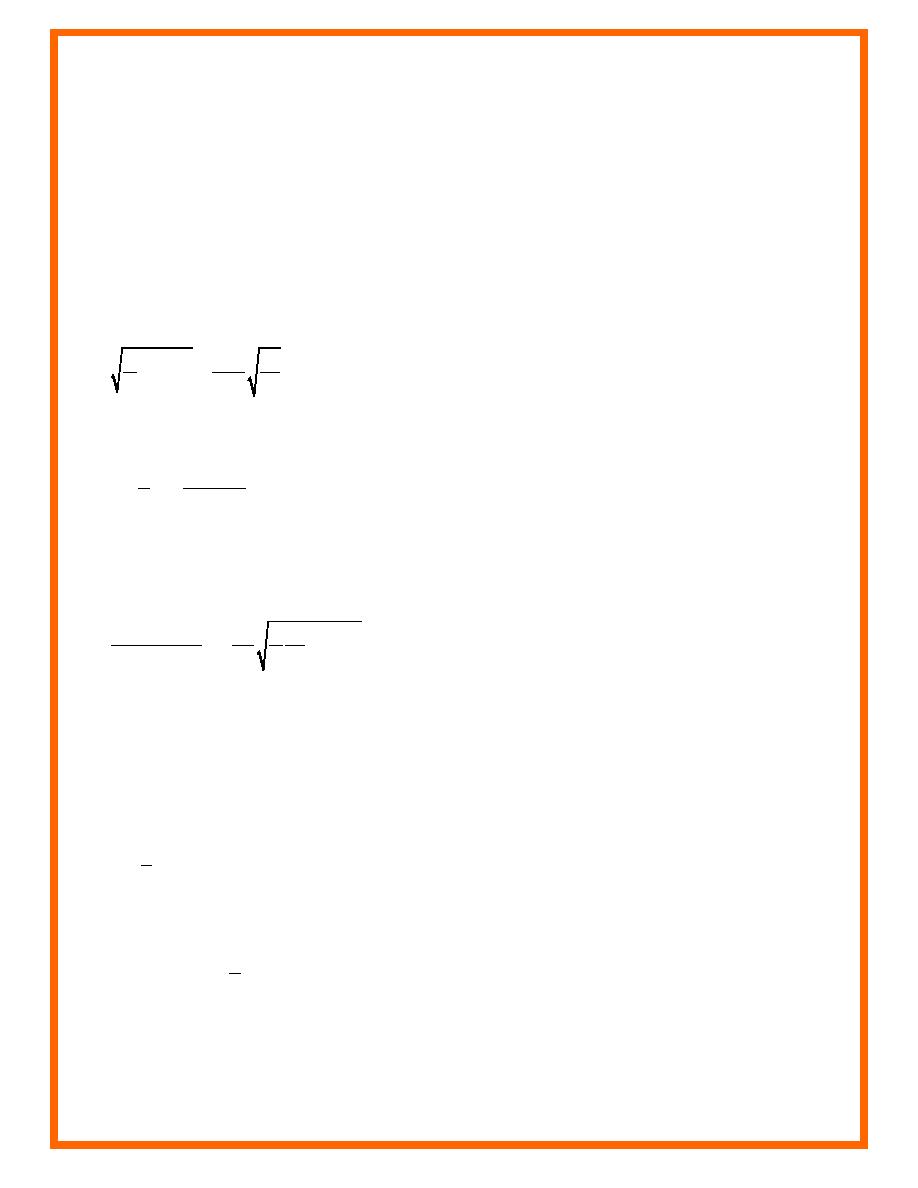
C gr + U cos(δ - α ) = 0
(13)
If Equation 13 is fulfilled, the denominator in Equation 9 becomes 0 and the wave rays (along
which the energy is conserved) are perpendicular to the wave orthogonals. At the point of
blocking, the wavelength attains its minimum value, which may be estimated from:
d
1
d
tanh kd =
(14)
1- n
L
Lo
where
1
2kd
n = 1 +
(15)
2 sinh 2kd
The required blocking speed associated with Equation 14 may be estimated from Equation 13,
once the wavelength L at blocking has been determined for a specific Lo and d. This criterion
may be written in non-dimensional form as:
U cos(δ - α)
n Ld
=-
tanh kd
(16)
2π d Lo
gTa
Thus, for a specific ratio d/Lo the required blocking speed might be determined from
Equations 14 and 16. Figure 2 displays the non-dimensional blocking speed as a function of
d/Lo.
Asymptotic solutions to the conditions for blocking may be readily obtained for shallow and
deep water. In deep water, that is, kd → ∞, Equation 14 yields:
1
L=
Lo
(17)
4
and Equation 16 produces:
1
U cos(δ - α) = - Co
(18)
4
5


 Previous Page
Previous Page
