ERDC/CHL CETN-IV-25
June 2000
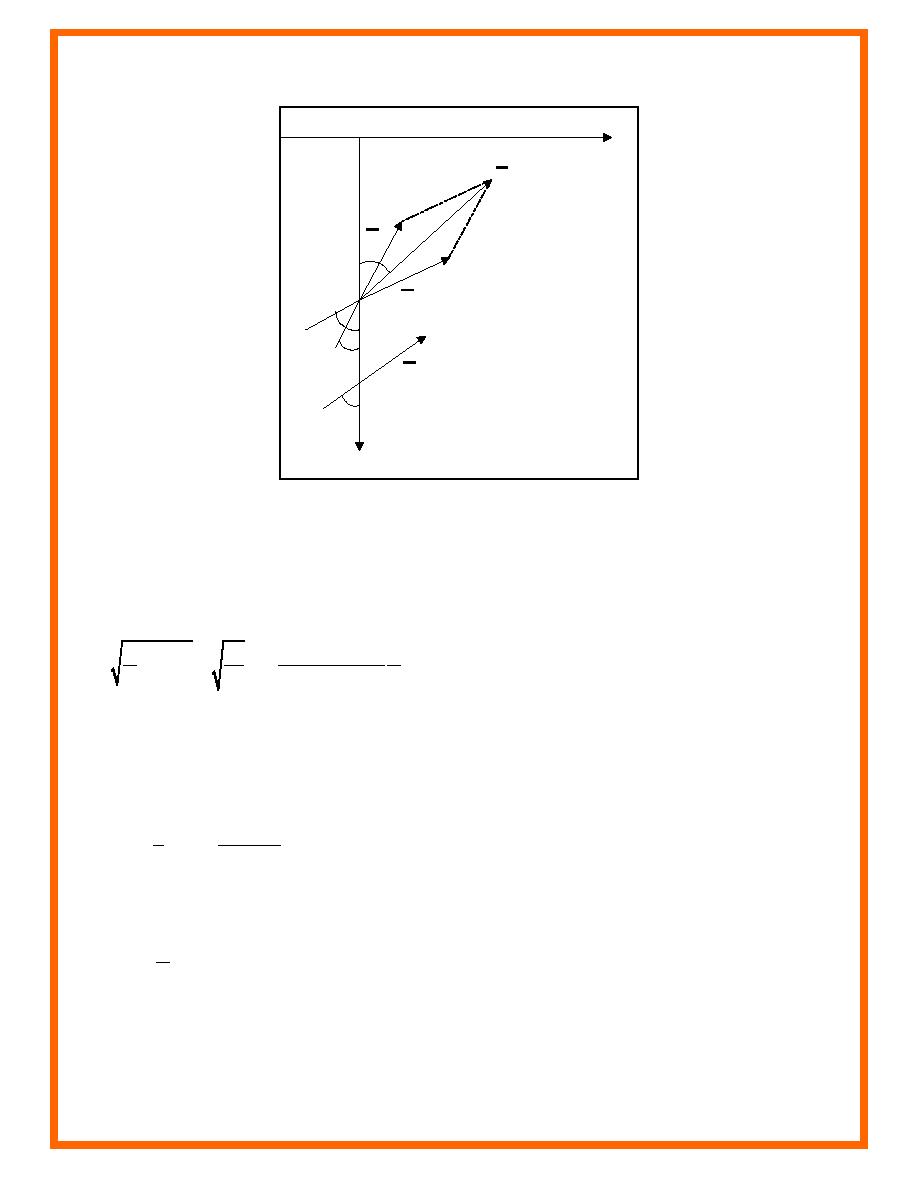
Longshore D stance
y
Cga
Cgr
β
U
δ
α
W
ϕ
Cross-Shore Distance
x
Figure 1. Definition sketch for waves propagating
on a current (overbar implies a vector)
To determine the wave properties at a certain total depth d = h + η, where h is the still-water
relationship including a current has to be solved (Jonsson, Skougaard, and Wang 1970):
U cos(δ - α)Ta d
d
d
tanh kd =
1 -
(4)
L
L
Lo
d
in which Ta is the absolute wave period, L the wavelength at depth d, k the wave number
(2π/L),and Lo denotes the deepwater wavelength neglecting the current (=gTa2/2π). Equation 4 is
solved by a Newton-Raphson technique, which gives rapid convergence. The relative group
speed is determined from:
1
2kd
Cgr = Cr 1 +
(5)
2 sinh 2kd
and the relative phase speed Cr is computed as:
1/ 2
g
Cr = tanh kd
(6)
k
in which g is the acceleration of gravity. As can be seen from Equation 4, the wave angle α must
be known before the wave properties can be calculated. Thus, Snell's law is employed to
3


 Previous Page
Previous Page
