ERDC/CHL CETN-IV-25
June 2000
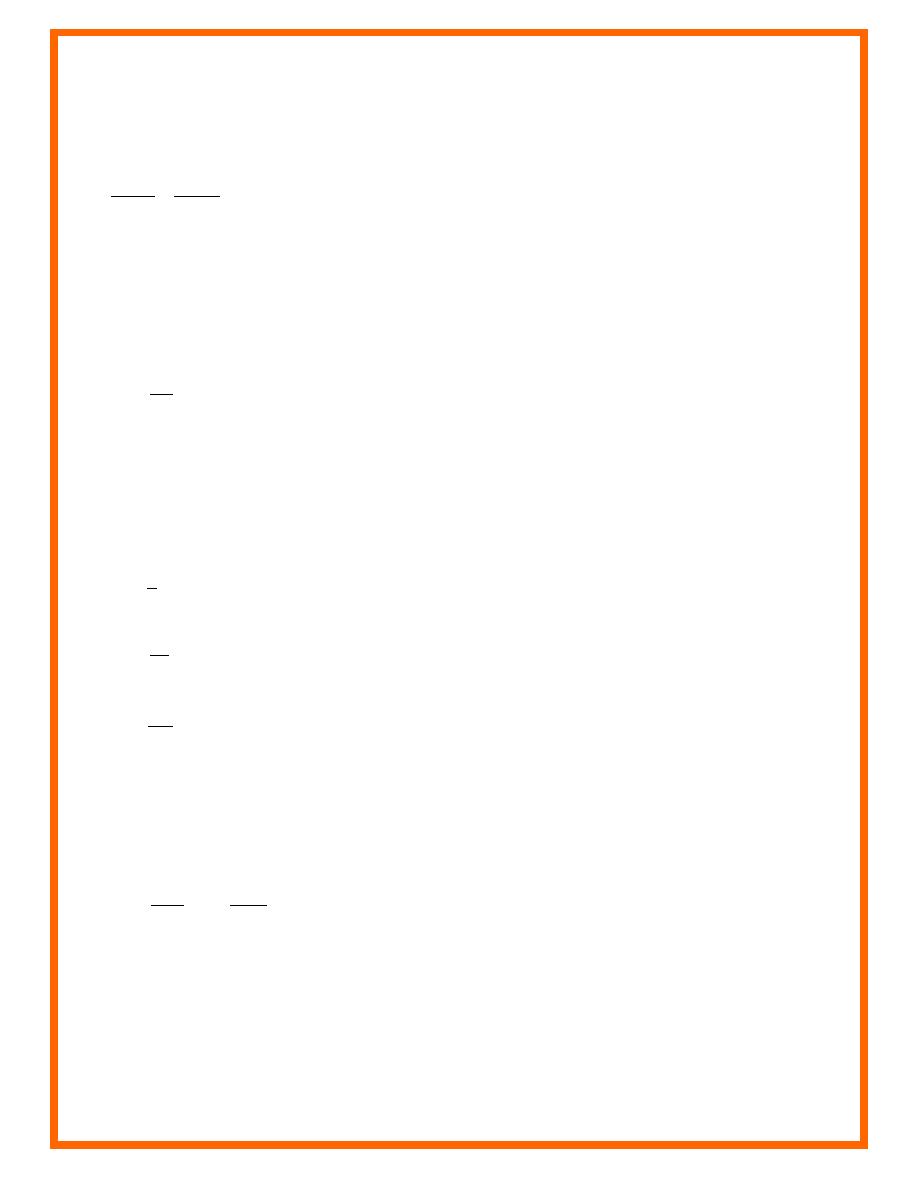
determine wave refraction and the variation in α across the profile. For a wave traveling
between locations denoted by indices 1 and 2, Snell's law is written:
sin α1 sin α 2
=
(7)
L1
L2
Equations 4 and 7 must be solved simultaneously because both α and L are unknown at the next
grid point in the numerical calculations.
The wave energy dissipation in Equation 1 is given by (compare Smith, Resio, and Vincent
1997):
κ
ED =
( E - Es )Cgr
(8)
dD
where κ is an empirical coefficient (= 0.15), dD the length scale controlling the dissipation (equal
to the water depth d in Dally, Dean, and Dalrymple (1985)), and Es the energy of a stable wave
for which breaking ceases and a wave can reform. To generalize Equation 8 to describe all water
depths and both depth- and steepness-limited breaking, the following expressions are employed
for the stable wave energy and the dissipation length scale:
1
Es = ρgH s2
(9)
8
Γ
Hs =
Hb
(10)
γb
Hb
dD =
(11)
γb
where ρ is the density of water, Γ is an empirical coefficient (= 0.4), and γb is the ratio between
wave height and water depth at incipient breaking under depth-limiting conditions (typically
taken to be 0.78). The wave height at incipient breaking is calculated from the Miche criterion,
modified by Battjes and Janssen (1978) to be applicable for all water depths:
γ kd
0.88
Hb =
tanh b
(12)
0.88
k
In shallow water, Equation 12 reduces to Hb = γbd, indicating that Equations 8-11 recover the
original formulation by Dally, Dean, and Dalrymple (1985). Equation 12 is applied with the
local water depth to determine Hb from which Hs is obtained from Equation 10.
WAVE BLOCKING: Waves propagating on a current may experience blocking, if the current
is sufficiently strong and has a component opposing the waves. If blocking occurs, the wave
4


 Previous Page
Previous Page
