ERDC/CHL CHETN-III-67
September 2003
and
⎡
⎛ H ⎞⎤
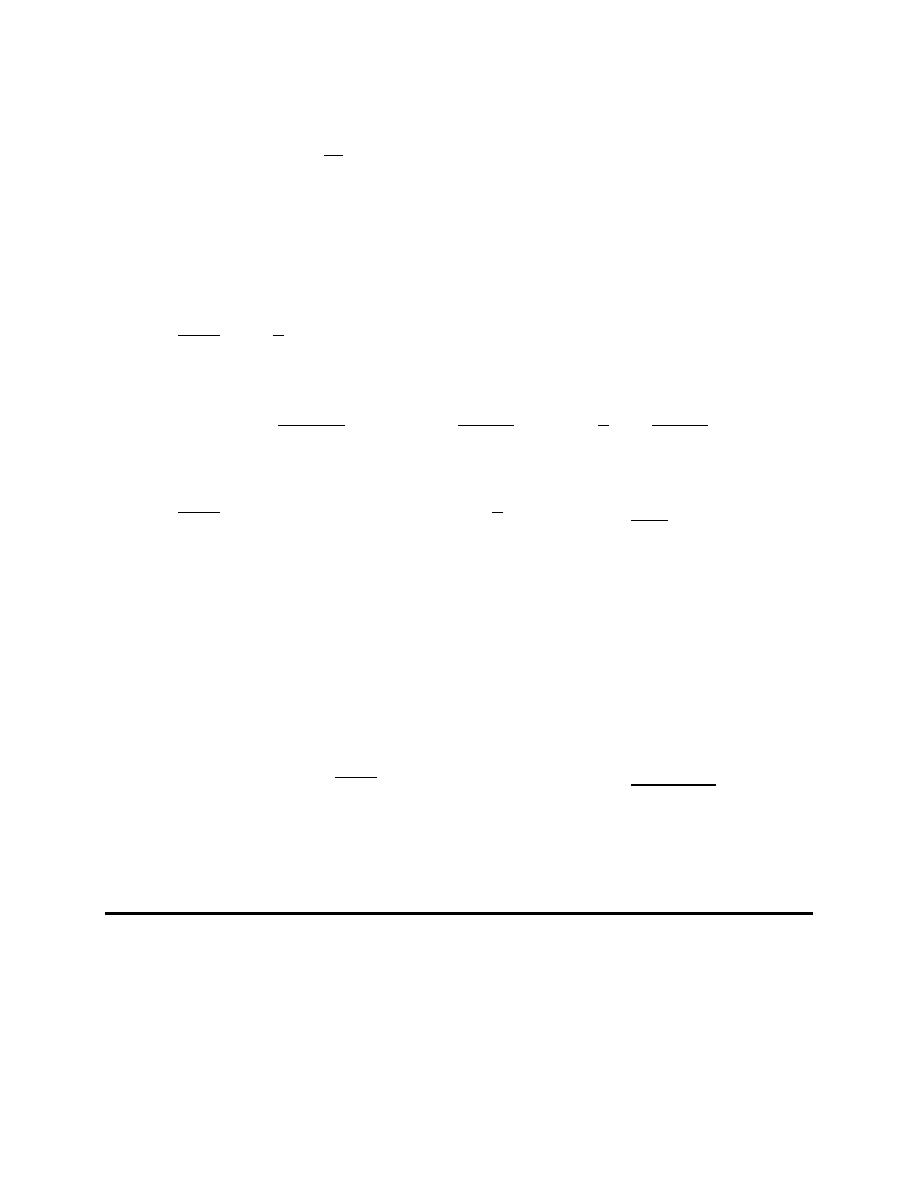
N = 0.69 tanh ⎢2.38 ⎜ ⎟⎥ = 0.69 tanh [2.38 (0.6)] = 0.615
⎝ h ⎠⎦
⎣
A quick check of the plots in Figure 3 with a value of H/h = 0.6 confirms the calculations.
Substituting values for H/h, M, and N into Equation 8 yields the nondimensional wave momentum
flux parameter for the specified solitary wave, i.e.,
⎛ MF ⎞
1
= ⎡ ( 0.6) + 2 ( 0.6)⎤
2
⎜
2⎟
⎝ ρ gh ⎠max 2 ⎣
⎦
⎧ ⎡ ( 0.923)
⎤ 1 3 ⎡ ( 0.923)
( 0.615)2 0.6 + 1
⎤⎫
⎪
( 0.6 + 1)⎥ ⎪
(
)
( 0.6 + 1)⎥ + tan ⎢
+
tan ⎢
⎨
⎬
2 ( 0.923)
⎪ ⎣ 2
3
2
⎪
⎦
⎣
⎦⎭
⎩
⎛ MF ⎞
⎡
⎤
1
tan ( 0.7384) + tan3 ( 0.7384)⎥ = 1.161
= 0.78 + 0.3278
⎜
⎟
⎢
ρ gh2 ⎠max
3
⎣
⎦
⎝
The plot shown in Figure 4 agrees with the previous calculation for a value of H/h = 0.6.
Caution: If you perform the previous calculation on a hand-held calculator, you may need to
convert the arguments of the tangent function from radians to degrees before taking the tangent.
Calculate the Maximum Depth-Integrated Wave Momentum Flux: Just as for the regular wave
example, the dimensional value of maximum depth-integrated wave momentum flux per unit length
along the wave crest is calculated as
⎛ MF ⎞
(MF)max = (ρg) (h2)
= (64 lb/ft3) (15 ft)2 (1.161) = 16,718 lb/ft
⎜
2⎟
⎝ ρ gh ⎠max
The value of (MF)max for the solitary wave is 1.74 times greater than the regular wave having the
same value of H/h. In fact, the solitary wave is the limit of the regular wave as wave period becomes
very long.
SUMMARY: This CHETN has described a new parameter representing the maximum depth-
integrated wave momentum flux occurring in a wave. Because wave momentum flux has units of
force per unit crest width, it is a physical descriptor of wave forces acting on coastal structures. For
periodic waves an easily applied empirical expression was given to estimate nondimensional
maximum depth-integrated wave momentum flux as a function of relative wave height and relative
11


 Previous Page
Previous Page
