ERDC/CHL CHETN-IV-34
June 2001
hW V
1 - exp -λ c 2 f
q (∆z) 1 - exp(-λ∆z / ha )
ha U a
p = in
=
=
(7)
1 - exp(-λ)
1 - exp(-λ)
qin (ha )
With the above expressions, p, which can be called a "trapping ratio," can be estimated from the
idealized geometry of the channel (channel width and average depth at the particular time of the
calculation), sediment fall speed, upstream current speed and depth, and decay coefficient λ of
the concentration profile.
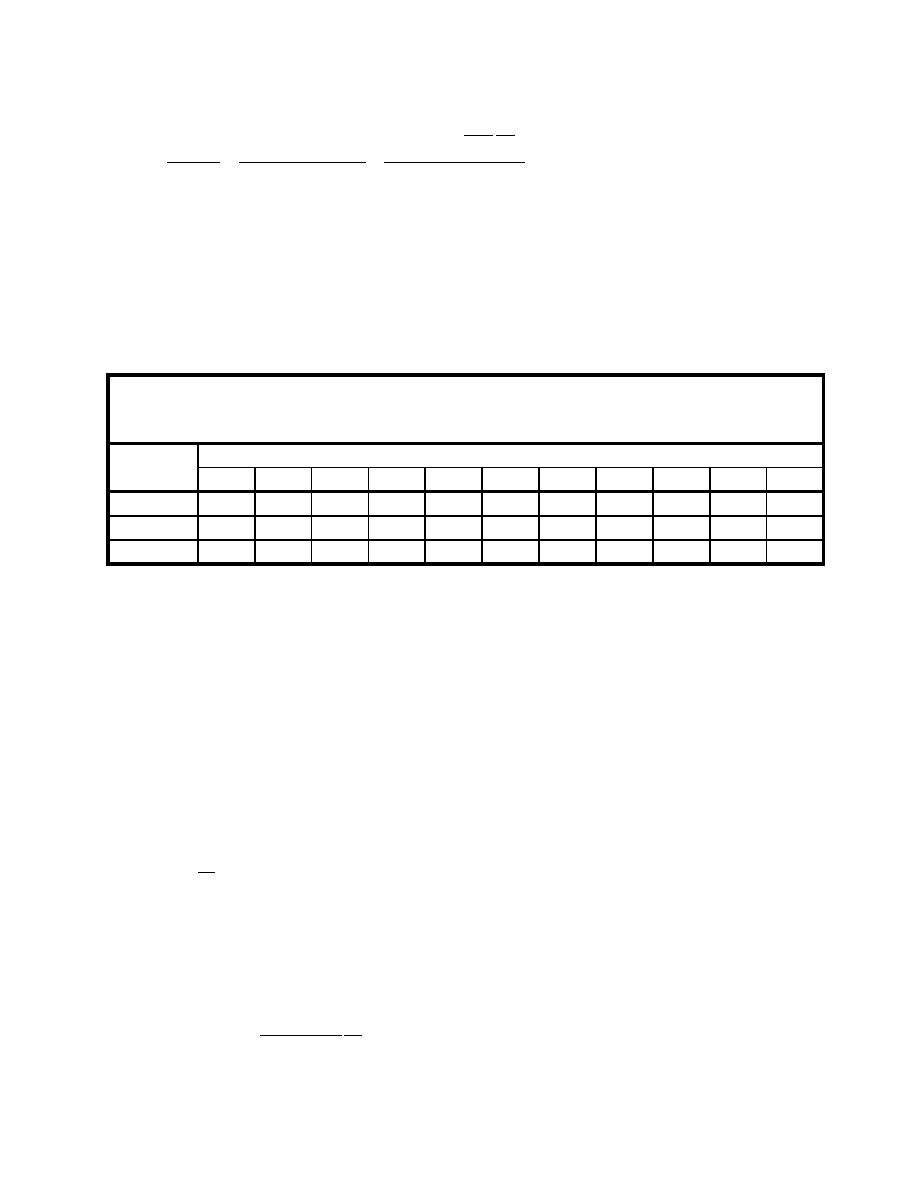
Table 1 lists sediment fall speeds for quartz grains of typical diameters and for different water
temperatures, which will change water viscosity and, therefore, sediment fall speed.
Table 1
Selected Values of Fall Speeds (m/s) for Quartz Sand Particles of Specified Diameters
(after Hallermeier (1981))
Grain Size, mm
Temp.
deg C
0.15
0.20
0.25
0.30
0.35
0.40
0.50
0.60
0.80
1.00
2.00
10
0.015
0.023
0.029
0.035
0.042
0.048
0.062
0.075
0.104
0.132
0.189
20
0.018
0.025
0.032
0.039
0.046
0.054
0.069
0.084
0.115
0.133
0.189
30
0.020
0.027
0.035
0.043
0.051
0.059
0.075
0.092
0/119
0/133
0.189
Suspended Sediment Decay Coefficient: The decay coefficient λ has been measured to
have a representative value of 1.65 with standard deviation of 0.68 for surf-zone sand with
median grain size in the range of 0.14 to 0.22 mm (Kraus and Dean 1987). Because λ is grain-
size dependent, in this section a rational method is presented for extending the result to other
grain sizes.
If the time-averaged turbulence intensity is homogeneous through the water column, which is a
reasonable representation of a surf zone or area of breaking waves, it is known that the vertical
distribution of the sediment concentration is proportional to the quantity exp[-(V f / εs ) z] , where
εs = sediment-mixing coefficient. Adopting concepts of Battjes (1975), the sediment-mixing
coefficient can be estimated as,
1/ 3
D
εs = kd
ha
(8)
ρ
where kd = dimensionless empirical coefficient expected to be independent of grain size, D =
energy dissipation produced by the breaking waves, and ρ = density of water. The standard
z
Vf
C ( z) = Cb exp -
(9)
kd ( D / ρ)1/ 3 ha
4


 Previous Page
Previous Page
