CETN IV-18
March 1999
Alternately, a value for qe can be read directly from the plot in Figure 2 by finding the depth of
9.5 m on the vertical axis, extending a horizontal line to intersect with the "de = 0.2 mm" line,
and reading the corresponding discharge on the horizontal axis. Figure 2 gives a value of
qe ≈ 10.5 m 2 / s
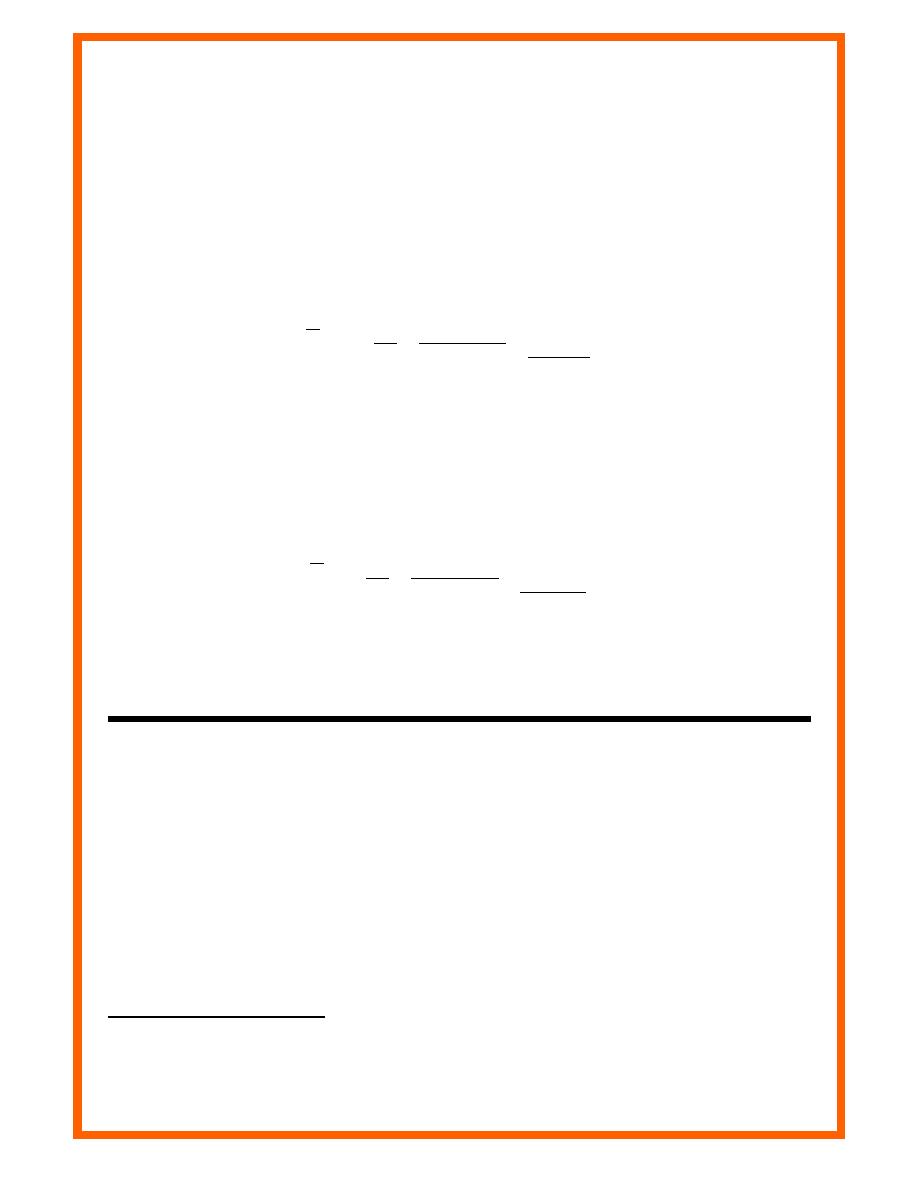
The maximum mean velocity corresponding to the equilibirum discharge is found from
Equation 5 as
qe 10.5 m 2 / s
=
=
= 1.1m / s
V scour
9.5 m
h
The same result could have been read directly from the curve in Figure 3 corresponding to de =
0.2 mm. Also note these estimates assume maximum discharge occurring at mllw.
Once the scour hole was filled in and capped at the -4.5-m mllw elevation, a similar storm
producing the same discharge through the gap between the North Jetty and Detached Breakwater
will produce an increased mean velocity given by
qe 10.5 m 2 / s
=
=
= 2.4 m / s
V sill
4.5 m
h
Visual estimates of flow speed through the gap during a storm after the sill was placed were on
the order of 2.5-3.0 m/s.1 The estimate of increased mean velocity is useful for determining the
absolute minimum scour blanket stone size if scour holes are filled and covered over with stone.
Example 2: Freshwater Discharge. A recent modification to the jetty system of a fictitious
tidal inlet on the Pacific Coast resulted in ebb-flow redirection and the formation of a 6-m-deep
scour hole adjacent to one of the jetties. The bed material is quartz sand with de . 0.6 mm
(0.0006 m). The scour hole in its present configuration does not threaten the jetty toe.
During normal conditions, only minor freshwater runoff empties into the bay and flows out the
entrance channel. However, during El Nio years, a large quantity of freshwater runoff flows
into the bay via flood channels. It is estimated that the maximum freshwater runoff will increase
the discharge per unit width through the inlet throat by 3 m2/s for a period lasting several days.
What will be the maximum depth of the scour hole as a result of the freshwater
surcharge?
1
Personal Communication, 1998, B. R. Schwichtenberg, U.S. Army Engineer District,
Los Angeles, Los Angeles, CA.
9


 Previous Page
Previous Page
