APPLICATION OF THE EQUILIBRIUM DISCHARGE DEPTH RELATIONSHIP:

cetniv-18
Page Navigation
5

CETN IV-18

March 1999

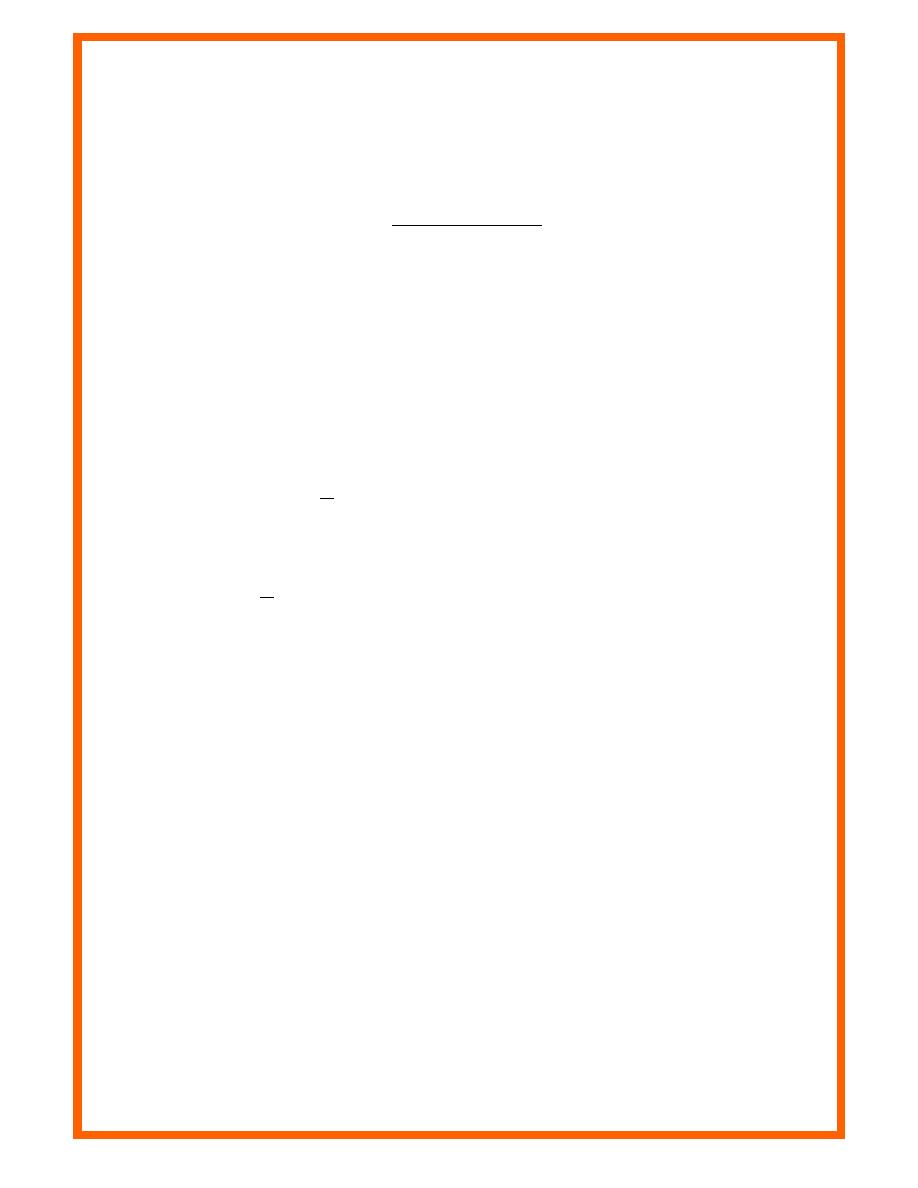
For a given noncohesive sediment, there is an equilibrium scour depth, he, associated with the

equilibrium discharge qe. The depth he is taken relative to the tide level at maximum discharge.

An expression for he is obtained by rearranging Equation 6 to get

0.234 qe / 9

he =

(7)

[g (S s - 1)]

4/9

1/ 3

Although it might be possible to have depths greater than the equilibrium scour depth, these

depths would have to be caused by some process other than the maximum discharge at that

location. Estimates of equilibrium scour depth from Equation 7 should be considered

conservative because the estimates represent the outer envelope of the field data. In reality, the

maximum discharge per unit width may not persist long enough to allow scoured depths to reach

the predicted equilibrium depth.

Finally, substitution of the value of Ce into Equation 3 and rearranging provides a relationship for

mean velocity at a location in terms of the equilibrium depth and sand parameters, i.e.,

V = 5.12 [g (S s - 1)]

1/ 2

d e3 / 8 he / 8

(8)

Plots of Equations 7 and 8 for a variety of quartz sand sizes are given in Figures 2 and 3. These

plots show equilibrium depth (he) as a function of equilibrium discharge per unit width (qe) and

mean flow velocity ( V ), respectively, for a range of quartz sand median grain-size diameters.

The plots illustrate the effect of grain-size diameter on the equilibrium depth. As expected,

channels with coarser sand have less depth at equilibrium under the same flow condition.

APPLICATION OF THE EQUILIBRIUM DISCHARGE DEPTH RELATIONSHIP: The

semiempirical relationships for equilibrium depth as a function of sand parameters and discharge

per unit width (Equation 7) or mean velocity (Equation 8) give depth estimates that are probably

conservative, i.e., deeper than might actually occur for the specified discharge. Use of these

formulas should be restricted to regions in the inlet throat where the scour appears to be caused

by the maximum discharge. For example, depths in scour holes formed by vortices associated

with flow separation will not be predicted by the equilibrium discharge depth relationship. In

addition, the equations do not account for depth increases because of wave action in the channel.

Important Note: Correct use of the predictive equations in this CETN requires that all

variables be given in a consistent set of units. In particular, sediment grain size needs to be

expressed in the same length unit used for qe and g in the equations (meters in the following

examples).