ERDC/CHL CHETN-III-70
July 2005
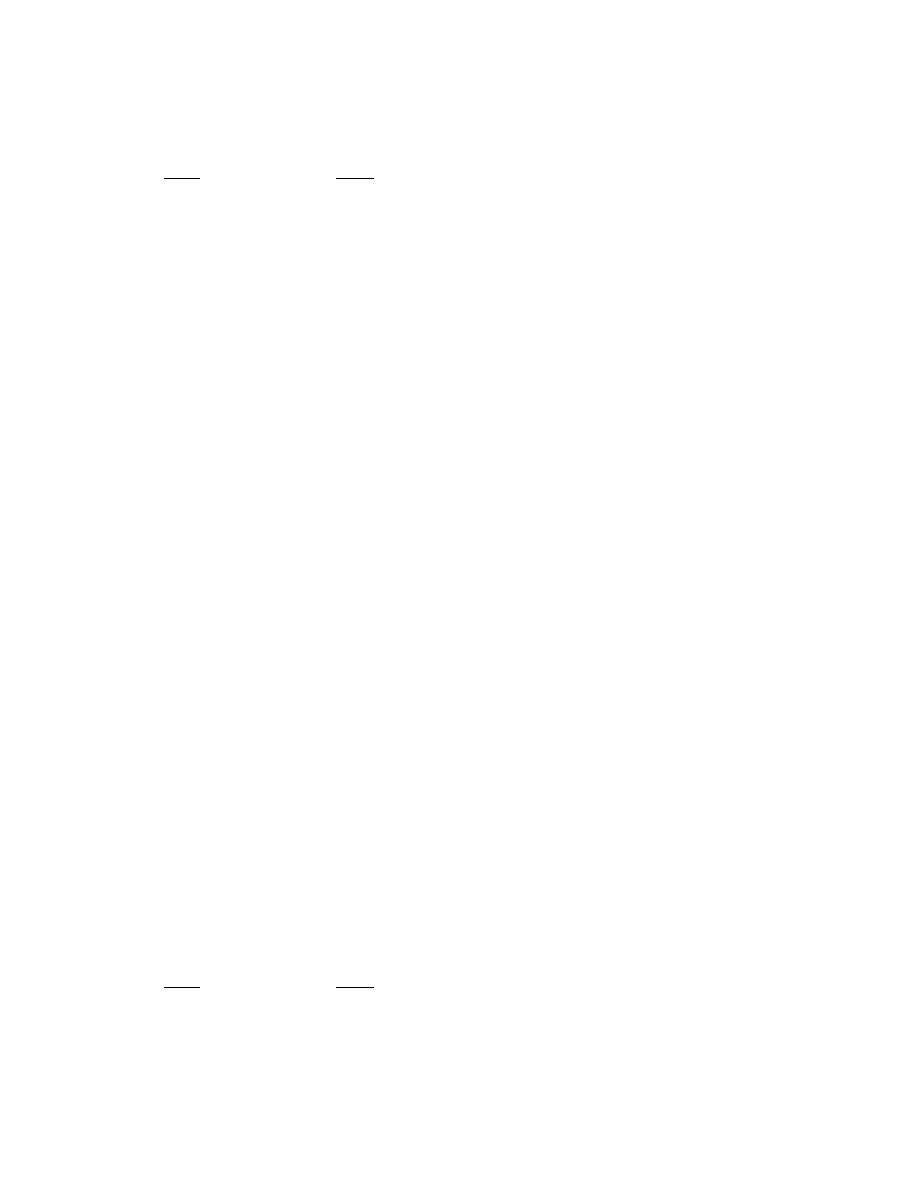
Newer equation for breaking (plunging/spilling) waves (Hmo/Lp > 0.0225):
1/ 2
0.7 ⎛ M
⎞
Ru 2%
= 4.4 ( tan α ) ⎜ F2 ⎟
1.5 ≤ cot α ≤ 30
for
(12)
⎝ ρgh ⎠
h
Equation 12 covers a much broader range of slopes, but it is slightly less accurate than Equation 11
when applied to structure slopes in the range 1.5 ≤ cot α ≤ 4.0. Nevertheless, having one equation
that predicts irregular wave runup over such a wide range of slopes is convenient, and it supports the
simple concept used to derive the runup equation.
Irregular Wave Runup Prediction for Rough, Impermeable Slopes: Slope roughness will
reduce the 2-percent runup level predicted using the equations for smooth, impermeable slopes
(Equations 10 and 11). One engineering approach is to multiply the runup estimates for smooth,
impermeable slopes by a reduction factor to account for various types of slope roughness. The
Coastal Engineering Manual contains reduction factors as summarized by de Waal and
van der Meer (1992) based on Dutch experience, and the reduction factor for rock and riprap
structures (1 and 2 layers) varies between 0.5 and 0.6. Rock and riprap structures impede runup not
only by slope roughness, but also by permeability of the riprap and any underlayers placed over the
impermeable slope. Voids are constantly filling and draining with the wave runup/rundown cycle,
and the effect of riprap permeability will vary with wave period. Thus, using a single constant to
represent runup reduction associated with rough, impermeable riprap slopes is simplistic. However,
this approach is justified by the success of past runup estimation formulas.
The original riprap slope runup data of van der Meer and Stam (1992) and Ahrens and Heimbaugh
(1988) were plotted as a function of the wave momentum flux parameter to ascertain any difference
between breaking (plunging and spilling) waves on the slope and nonbreaking (surging and
collapsing) waves. No strong trend was evident for rough slopes, whereas a distinct difference was
apparent for runup on smooth slopes (Hughes 2003b, 2004b). Because the majority of the laboratory
data represented waves that break on the slopes, the newer runup equation (Equation 12) for
plunging/spilling waves was used to estimate an appropriate reduction factor for runup on rough,
impermeable slopes. This method is appealing because it retains the conceptual runup model shown
in Figure 2. The best-fit reduction factor representing the mean of the data was 0.505, which is at the
lower end of the reduction factor range for rock-armored slopes given by de Waal and van der Meer
(1992).
Applying the reduction factor to Equation 12 results in the following new equation for estimating
irregular wave runup on rough (riprap) impermeable slopes for both breaking and nonbreaking
incident wave conditions.
New equation for irregular wave runup on rough, impermeable slopes:
1/ 2
0.7 ⎛ M
⎞
Ru 2%
= 4.4 ( tan α ) ⎜ F2 ⎟
2.0 ≤ cot α ≤ 4.0
(0.505)
for
(13)
⎝ ρgh ⎠
h
Figure 3 compares estimated values of relative runup (Ru2%/h) to measured values. Reasonable
correspondence was expected because these were the same data used to determine the appropriate
6


 Previous Page
Previous Page
