Table 1. Rates of Volume Change for Shinnecock Inlet Sediment Budget, 1938 to 1979 (thousands of cu m/year)

cetniv-15
Page Navigation
13

CETN IV-15

Rev. September 1999

760

∆ysl _ A1 = ∆ysl _ A2 =

(0.003) = 0.22 m/year

B + Dc

3 + 7.5

or,

Qsl _ A1 = Qsl _ A2 = (∆ysl _ A1

or ∆ysl _ A2)(∆x) ( DA) = (0.22 m/year) (3,200 m) (10.5 m) ~ 7,300 cu m/year

The total change in volume for the inlet channel and shoals was given as 111,000 cu m/year. To

fully develop the inlet sediment budget, this quantity will be proportioned between the ebb shoal,

inlet channel, and flood shoal following the conceptual budget as guidance. Table 1 lists the rate

of measured volume change ∆V, beach fill placed P, dredging (removal) R, and losses because of

relative sea-level rise Qsl for A1, each region of the inlet, and A2.

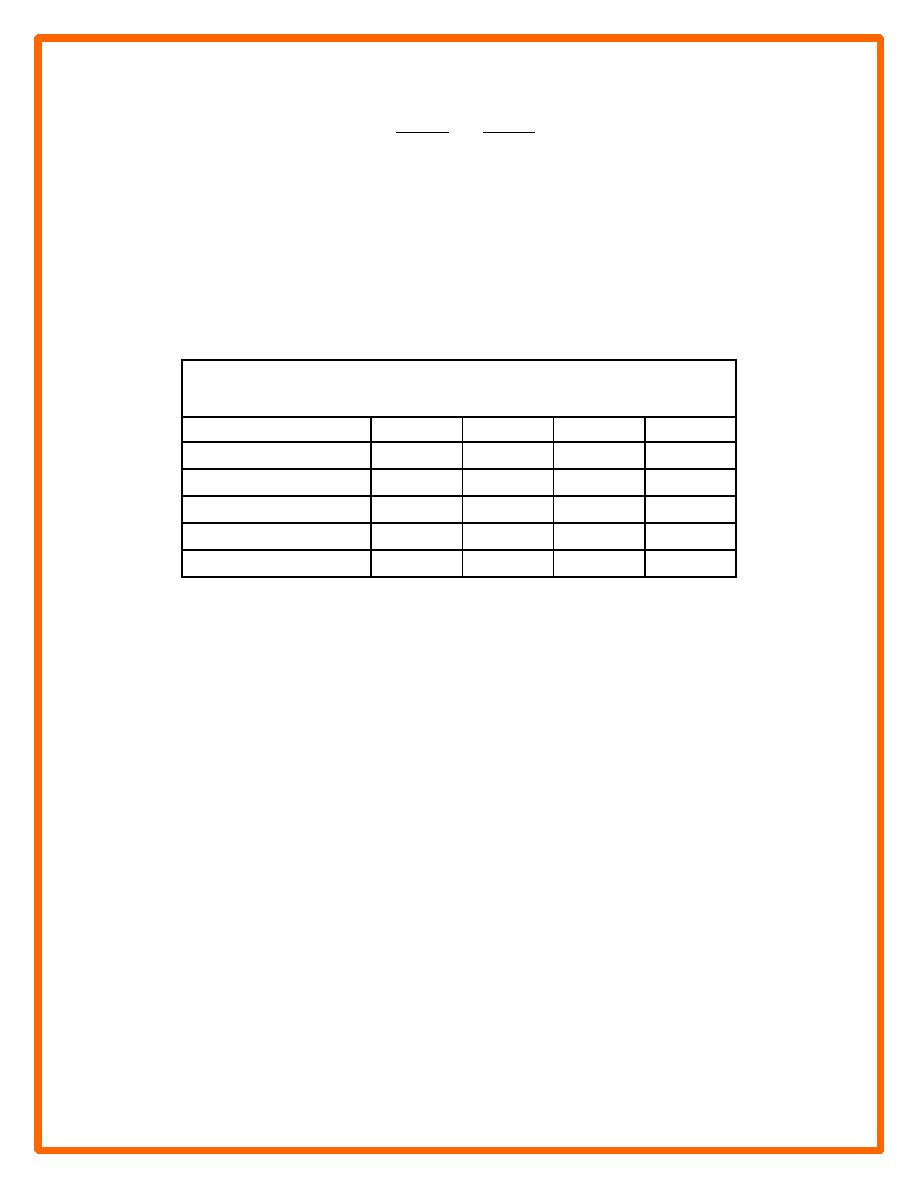
Table 1

Rates of Volume Change for Shinnecock Inlet Sediment Budget,

1938 to 1979 (thousands of cu m/year)

Control Volume

∆V

Qsl

A1 (Adjacent Beach 1)

7.3

Inlet: Ebb Shoal

Inlet: Channel

2.4

Inlet: Flood Shoal

A2 (Adjacent Beach 2)

-48

7.3

Refining Conceptual Sediment Budget. To formulate the inlet sediment budget, one can assume

a rate of net transport at the updrift boundary, Qnet_A1 = 230,000 cu m/year. This value is within

the range identified in the conceptual sediment budget. In a more expanded analysis than

presented here, a range of values for Qnet_A1 can be applied in the sediment budget to examine

fully the sensitivity of the inlet sediment-transport magnitudes and pathways to this parameter.

The ratio of QR and QL was given as 1.9, and entering this value into Equation (2) gives,

= Q R _ A1 - Q L _ A1 = 1 .9 Q L _ A1 - Q L _ A1 = 0 .9 Q L _ A1

Q net

_ A1

230 = 0 .9 Q L _ A1

Q L _ A1 = 255 and Q R _ A1 = 485

Considering the entire reach as the control volume forms a macrobudget. Applying Equation 1

gives,